
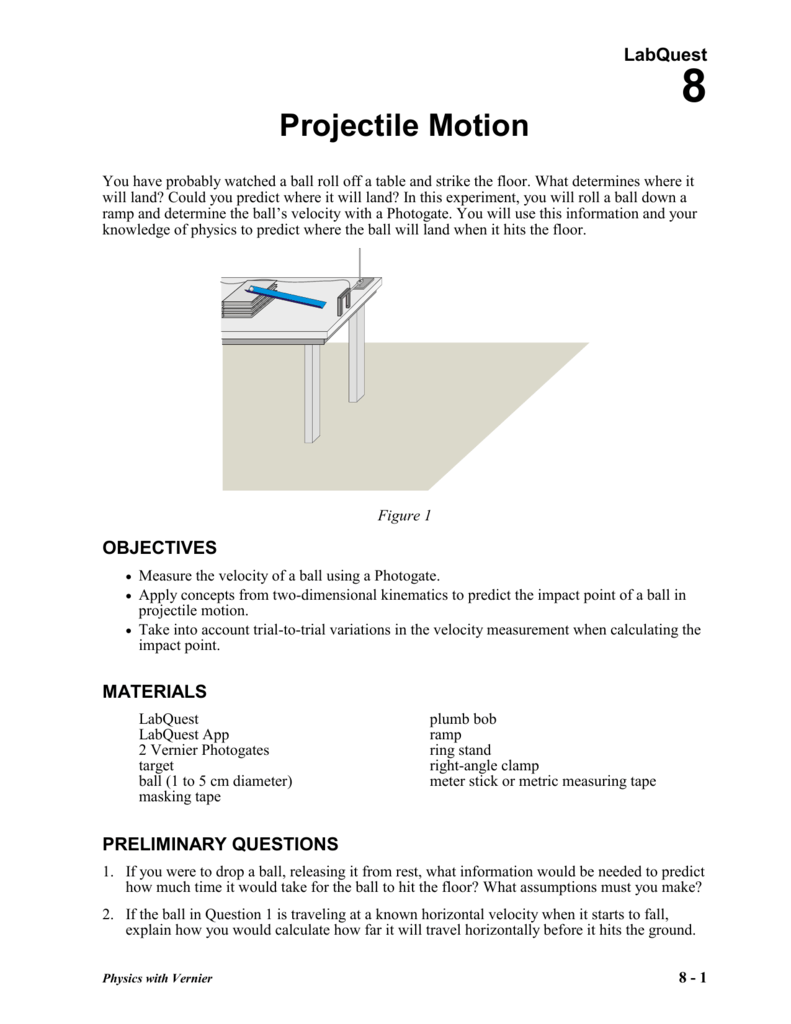
Describe two examples of projectile motion which you have observed or experienced outside of the physics lab. Ideally what mathematical curve is the trajectory? 10. Which sign do you use? What, if any, is the significance of the other sign? 9. (11) of the Theory section, there is a ± sign. How might you directly measure the initial velocity of the ball? What additional apparatus would you need? 8. 2 Projectile Motion the range equation in your text. Practice firing the gun the same way each time to minimize the variation in initial velocity. Adjust the spring tension so that the range, when fired horizontally, is between one and two meters. After the instructor has demonstrated the use of the gun, load it and fire a few practice shots. (Note: Do not load or fire the spring gun until its use has been described and demonstrated by your instructor) The angle of projection is measured with a protractor, and the initial velocity can be varied by adjusting the tension on the spring with the adjusting knob on the back of the gun.
1) allows both the angle of projection and the initial velocity to be varied. (4) to rewrite this in terms of quantities you will measure: y = h + xtan θ - x 2 g 2v 02cos2θ (9) Again, the range R is the value of x when y = 0 substituting this gives h + R tan θ - R 2 g 2v 02cos2θ = 0 (10) This is a quadratic equation in R solving for R we get the range equation, R = v 0 2cosθ g sin θ ± sin 2θ + 2hg v 02 (11) APPARATUS ❏ Spring gun set-up ❏ Spring gun ball ❏ Gun mounted on frame w/ protractor ❏ Bar level ❏ Hook collar w/ pointer ❏ Two-meterstick ❏ 2 Bench clamps ❏ Plumb-bob ❏ 2 Aluminum bar supports w/ wing nut ❏ Masking tape ❏ Short rod ❏ Paper EXPERIMENT The experiment consists of measuring the range R of a small ball fired from a spring gun at various angles from the vertical. (2) for t and substitute the result into Equation 3, (using xo = 0 and yo = h) we get y = h + v 0y v 0x x - g 2v 0x2 x 2 (8) We can use Eqs.

Now consider the case in which θ ≠ 0° (initial velocity is not horizontal). (6) becomes 2 2 2 0 ov gRh −= and solving for vo we get 1 Projectile Motion v 0 = R g 2h for the case θ = 0° (7) B.

2 2 2 ov gxhy −= (6) When the object hits the floor the x and y positions are x = R and y = 0. (2) and (3) become x = v 0t and y = h - 1 2gt 2 (5) If we eliminate t in Eqs.(5) we get y as a function of x. For the case in which θ = 0° (initial velocity is horizontal), Eqs. Hence, the vector equation (1) becomes two scalar equations: x = x0 + v 0xt (2) y = y0 + v 0yt - 1 2gt 2 (3) In terms of the angle θ, and the initial speed vo, the initial velocity components are (4) v 0x = v 0cosθ and v 0y = v 0sin θ A. The acceleration in the x direction is zero. If we neglect air resistance, the acceleration in the y direction is -g, due to gravity. Since the motion is in a plane, we need only look at the x and y components. THEORY For a given initial velocity, v, and initial position, 0 s0 ,the position of a particle, s, as a function of time, undergoing constant acceleration, a is given by r s = r s 0 + r v 0t + 1 2 r a t2 (1) This is a vector equation and can be broken up into its x, y, and z components. For further background information, refer to the sections in your textbook on projectile motion and motion with constant acceleration. For a given initial velocity, the projectile range will be measured for various initial angles, and also calculated by applying the theory for motion with constant acceleration. From the motion of a steel ball projected horizontally, the initial velocity of the ball can be determined from the measured range.
PROJECTILE MOTION LAB PDF
Download Projectile Motion Lab and more Physics Lab Reports in PDF only on Docsity!Projectile Motion The purpose of this lab is to study the properties of projectile motion.


 0 kommentar(er)
0 kommentar(er)
